The Value Investing framework of Warren Buffett's mentor allows for P/E ratios of 30 and more based on prevailing Interest Rates.
What Graham Wrote
Graham recommended a P/E ratio no higher than 13.3 based on an AA Bond Yield of 7.5%.
"Our basic recommendation is that the stock portfolio, when acquired, should have an overall earnings/price ratio—the reverse of the P/E ratio—at least as high as the current high-grade bond rate. This would mean a P/E ratio no higher than 13.3 against an AA bond yield of 7.5%."
Based on the same principle, a defensive investor in the U.S. today could consider stocks with P/E ratios up to 30, since 10-year AA Corporate Bond Yields are now close to 3.3%.
100 ÷ 7.5 = 13.3
100 ÷ 3.3 = 30
Some Quick Math
Adjusting Graham's framework to screen Defensive grade stocks with P/E ratios of 30 instead of 15 (all else being equal) would involve the following:
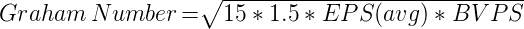
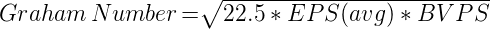
30 ÷ 15 = 2
√2 = 1.41
We would need to multiply the Graham Number of a stock by 1.41, to adjust it to a P/E of 30.
Note: Since the Graham Number is designed to balance Earnings and Assets, stocks with P/E values higher than 30 could clear Graham's rules too if they have lower P/B values.
Screening
a. Defensive
The filter values required on GrahamValue's screeners for finding Defensive grade stocks with the adjusted Graham Number would be:
Graham Number(%) ≥ 70%
Graham Number(%) is Graham Number ÷ Previous Close.
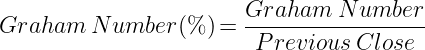
The reciprocal of 1.41 is 0.70, or 70%. So a stock with a Graham Number(%) of 70% or more will have a Previous Close that is 1.41 times its calculated Graham Number or less.
b. Enterprising
Prices for Enterprising grade stocks will have to be adjusted similarly.
Intrinsic Value is the price corresponding to a stock's Graham Grade: Defensive, Enterprising or NCAV. For Enterprising grade stocks, Intrinsic Value = Serenity Number.
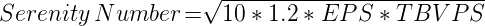
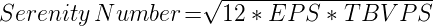
Intrinsic Value(%) is Intrinsic Value ÷ Previous Close, expressed as a percentage. GrahamValue's Advanced Graham Screener has a filter specifically for Intrinsic Value(%).

Considering current Interest Rates, Defensive and Enterprising grade U.S. stocks would need an Intrinsic Value(%) of 70% or higher to be classified as true Graham stocks today. This customization may differ for for non-U.S. economies.
Filter Calculator
The below JavaScript calculator can be used to automate the Yield to Filter calculation.
Yield Value (%)
Filter Value (%)
2022 Update
The US Corporate AA Effective Yield of 2.16% as of Jan 05 2022 would imply a Graham Number(%) and Intrinsic Value(%) of 57% for Defensive and Enterprising grade U.S. stocks, using a calculation identical to the one above. The filters on GrahamValue can accordingly be set to 55% or 60%, as per one's preference.
Note: Japanese stocks may similarly require the filters to be set to 20%.
c. NCAV (Net-Net)
NCAV grade stocks will require Intrinsic Values of 100% or higher. The NCAV Price is calculated based on asset values alone, which — unlike earnings yields — are not dependent on bond rates.
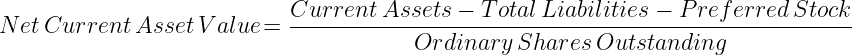
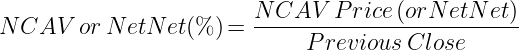
Watch Video
The below animated explainer video has been created with the updated Interest Rates for 2022 in mind.
Buffett Explains
Interest Rates ≈ Gravity
"Look at one of the two important variables that affect investment results: interest rates. These act on financial valuations the way gravity acts on matter: The higher the rate, the greater the downward pull... What an investor should pay today for a dollar to be received tomorrow can only be determined by first looking at the risk-free interest rate... People can see this easily in the case of bonds, whose value is normally affected only by interest rates. In the case of equities or real estate or farms or whatever, other very important variables are almost always at work, and that means the effect of interest rate changes is usually obscured. Nonetheless, the effect — like the invisible pull of gravity — is constantly there."
Intrinsic Value ∝ Bond Yields
Warren Buffett explains how the Intrinsic Value of a stock is dependent on prevailing Interest Rates and Bond Yields.
Gravity Again
In the longer version of the above video, Buffett can be seen referring to the gravity analogy again.
And Again
Here's Buffett explaining the gravity analogy again, but now in the context of Negative Rates.
Facebook Likes and Comments
Submitted by GrahamValue. Created on Monday 6th July 2015. Updated on Tuesday 1st August 2023.

Comments
Graham Number
Based on this and how the advanced screener calculates GN, should we be looking at 1.46*GN versus previous close when considering margin of safety from a dollars perspective?
70%
That's correct, LearningGraham!
The way to do that on Serenity's screeners is to screen for Defensive and Enterprising grade stocks with Intrinsic Value(%) greater than 70%.
Also, for Defensive grade stocks, Intrinsic Value = Graham Number.
Margin of Safety, Earning Yield vs Bond Yield
I believe Buffet said once that a good way to see the value of a stock is by comparing its earning yield vs 10 years treasury yield. If earning yield is at least 2x the current 10 years treasury yield it is a good buy. For example, 10 years treasury yield right now is 2.3%, which means if we buy a stock with P/E of 21.74 (4.6% earning yield), that's a good buy.
It is tough to find bargain nowadays. Dow hit all time high. S&P 500 index P/E ratio is 26.16.
Hundreds of Graham Stocks
Thank you for your informative comment, Will!
Serenity's screeners list hundreds of stocks that completely clear Graham's requirements as of today.
ICE BofA AA US Corporate Index Effective Yield
What is meant here is the return on share for all US companies? Like what we have in Saudi Arabia
https://www.saudiexchange.sa/Resources/Reports-v2/DailyFinancialIndicators_en.html
Which is now 2.18
Please Elaborate
Dear Omar_H,
Could you please explain your query in more detail?
Thank you for your comment!
Does this site mean the
Does this site mean the return on share for all companies in America, or does it mean the return on corporate bonds The US Corporate AA Effective Yield of 2.16%
U.K. and U.K. Bond Yields
Dear Omar_H,
Thank you for your comment!
That is correct.
Investing in economies other than the U.S. is explained using the Bond Yields of the U.K.
Thank you again for your comment!
Look at this link, Saudi
Look at this link, Saudi Market Reports. I think you can get it out of here. I will be grateful to you. This is what is left for me
https://www.saudiexchange.sa/wps/portal/ [truncated]
Sukuk Yield?
Dear Omar_H,
Thank you for your comment!
The official government link and the Saudi Exchange link do not appear to have Yield information.
But the S&P Global High Yield Sukuk Index shows a Yield of 6.43%, and Cbonds shows a Sukuk Yield of 4.303%.
Please use your own judgment in choosing an appropriate Yield value.
Thank you again for your comment!